Course content

Quantum Physics is the underlying theory that makes the foundation of 3 of the fundamental forces of Nature. If we want to truly understand how most phenomena in the Universe actually work we eventually reach the quantum world. In other words, as we dig deeper into how things like mirrors work or how solar cells work or how we know the temperature of the entire universe, we eventually get to “because Quantum Physics!”. All electromagnetic phenomena and all subatomic phenomena need Quantum Physics. It is one of the most important scientific ideas humans have discovered. And yet, it is not usually discussed either in High School or even at the college level, partly because its full treatment involves advanced math. However, it is possible to discuss the ideas and concepts using graphs and web tools that have the math behind the scenes but that focus on having us understand what those ideas are saying and what they imply.

In this course, you will see how we came to realize that nature blurs the lines between particles and waves, that objects can be in two places at once, and that under certain conditions they can do the equivalent of passing right through a door (e.g. see the animation above)! Niels Bohr, one of the fathers of Quantum Physics, put it thus: “Those who are not shocked when they first come across quantum theory cannot possibly have understood it.”  After taking this course, you will have a deeper understanding of one of the most bizarre yet key ideas that has helped us to understand how our Universe works.

GE credits

Fulfills the Science and Engineering requirement.

Representative books

John Stoddard, “Quantum Physics For Beginners, Into the Light: The 4 Bizarre Discoveries You Must Know To Master Quantum Mechanics Fast, Revealed Step-By-Step (In Plain English)”, Independently Published.

Leon Lederman and Christopher Hill, “Quantum Physics for Poets”, Prometheus Books.

Lukas Neumeier and James Douglas, “Quantum Physics for Hippies”.

Graphical Example: Electron Quantum states.

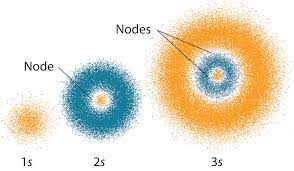
Quantum Physics requires us to abandon the idea of electron orbits, and instead think about regions of space where an electron has a higher probability of being found, as seen in the illustration above.  This is an example of how we can visualize what the equations of quantum mechanics say, without requiring solving the equations. We can look at graphs that contain the visual information of the solutions those equations, conveying the main findings of quantum theory, and helping us refine our picture of the universe.